NCERT Exemplar Class 8 Maths Chapter 1: Rational Numbers. NCERT Exemplar Solutions for Class 8 Maths Chapter 1 Rational Numbers prepare students for their Class 8 exams thoroughly.
Maths problems and solutions for the Class 8 pdf are provided here which are similar to the questions being asked in the previous year's board.
NCERT Exemplar Class 8 Maths Chapter 1: Rational Numbers
Class 8: Maths Chapter 1 solutions. Complete Class 8 Maths Chapter 1 Notes.
Main Concepts and Results
A number that can be expressed in the form p/q , where p and q are integers and q¹0, is called a rational number.
Lowest form of a rational number – A rational number p/ q is said to be in the lowest form or simplest form if p and q have no common factor other than 1 and q ¹ 0.
Addition, subtraction, multiplication and division of rational numbers are done in the same way as we do for fractions.
Rational numbers are closed under the operations of addition, subtraction and multiplication.
The operations of addition and multiplication for rational numbers are
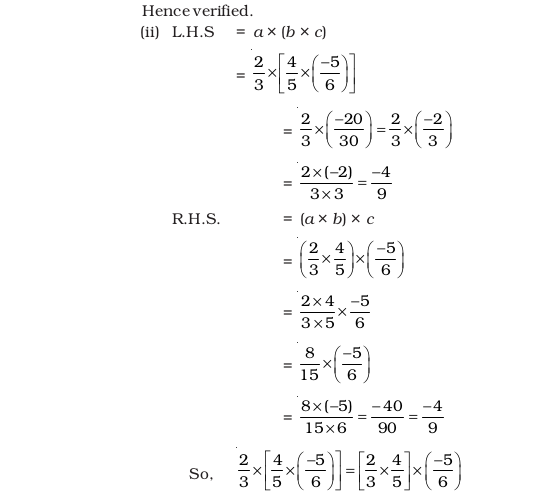
(i) commutative (ii) associative
The rational number 0 is the additive identity for rational numbers and the rational number 1 is the multiplicative identity for rational numbers.
The additive inverse of the rational number a /b is –a /b and vice-versa.
The reciprocal or multiplicative inverse of the rational number a b is
Distributivity of rational numbers – For all rational numbers a, b and c.
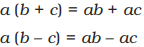
Rational numbers can be represented on a number line.
Between any two given rational numbers there are infinitely many rational numbers. The idea of mean helps us to find rational numbers between two given rational numbers.
Solved Examples
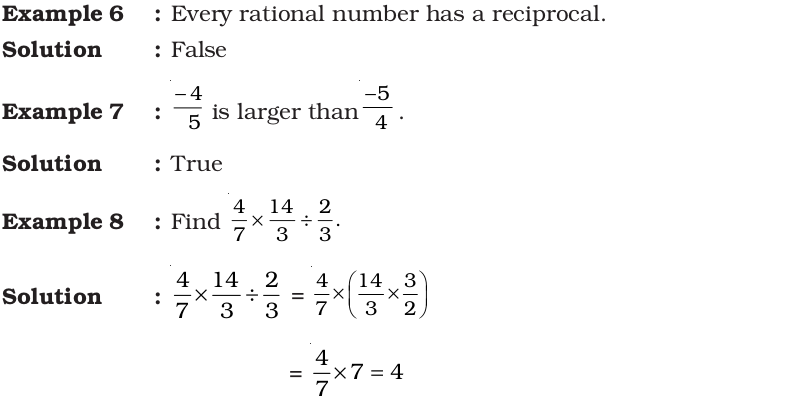
Example 1: Which of the following is not true?
Solution: The correct answer is (b).
Example 2: Multiplicative inverse of 0/1 is
(a) 1 (b) –1 (c) 0 (d) not defined
Solution: The correct answer is (d).
Example 3: Three rational numbers lying between −3/4 and 1/2 are
Solution: The correct answer is (c).
Fill in the Blanks Solved Examples
Example 4: The product of a non-zero rational number and its reciprocal is ________.
Solution : 1
Example 5: If x = 1 /3 and 6 7 y = 6 /7then xy −y/x = _______.
Solution : −16/7
Statements are true or false
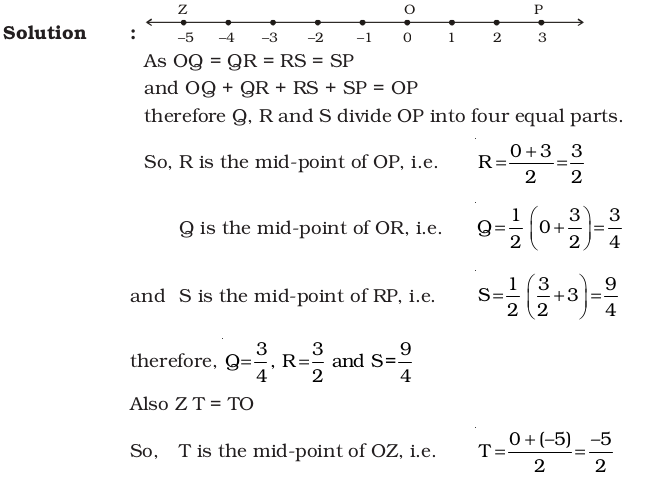
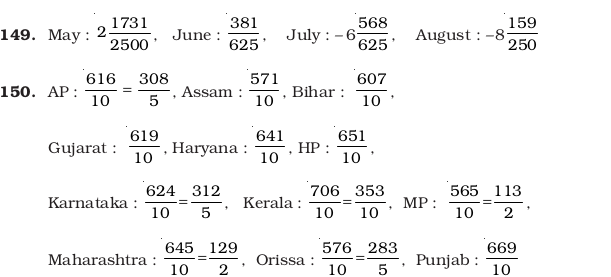
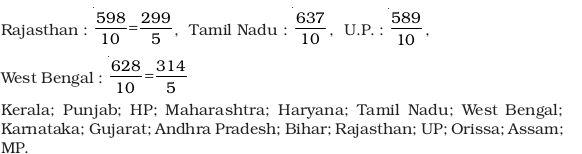
Example 10 : Let O, P and Z represent the numbers 0, 3 and -5 respectively on the number line. Points Q, R and S are between O and P such that OQ = QR = RS = SP. What are the rational numbers represented by the points Q, R and S. Next choose a point T between Z and O so that ZT = TO? Which rational number does T represent?
Think and Discuss
- Explain the first step in solving an addition equation with fractions having like denominators.
- Explain the first step in solving an addition equation with fractions having unlike denominators.
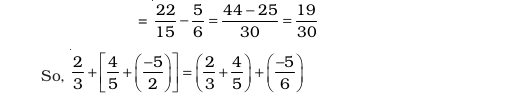
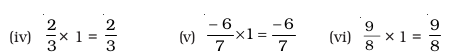
Observation
From (i) to (iii), we observe that: (i) When we add 0 to a rational number we get the same rational number. From (iv) to (vi), we observe that: (ii) When we multiply a rational number by 1 we get the same rational number. (iii) Therefore, 0 is the additive identity of rational numbers and 1 is the ‘multiplicative identity’ of rational numbers
Think and Discuss
Some other easier ways to find the answer. Is the product greater than both the rational numb or less than both the rational numbers?
Note Taking Skills
Focus on Graphic Organisers
You can use an information frame to organize information about a mathematical concept or property, such as the commutative property of addition.
Multiple Choice Questions
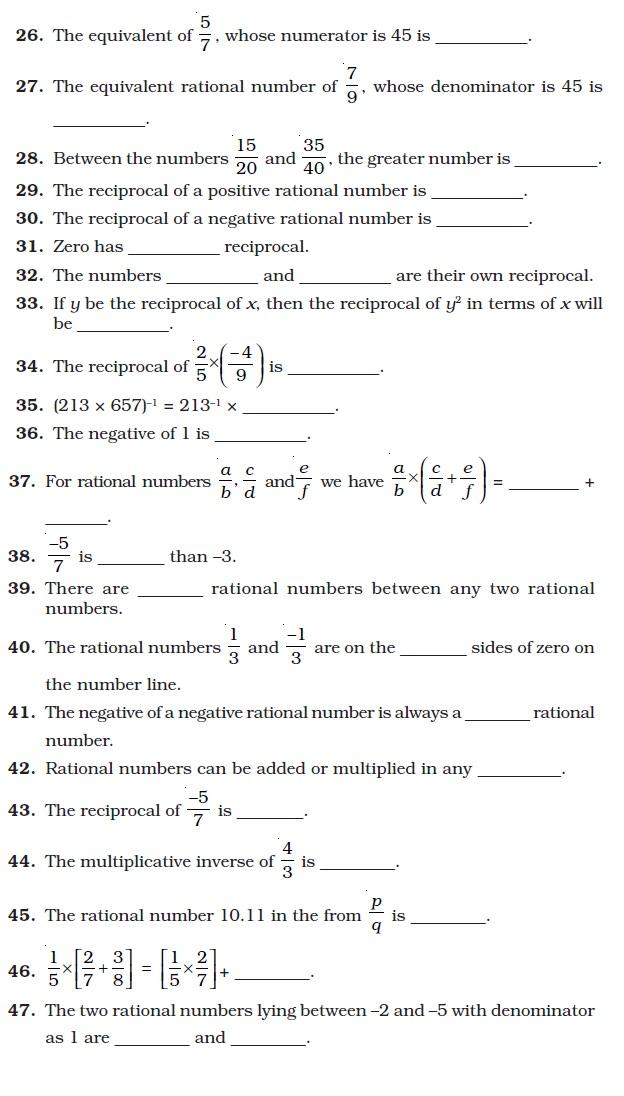
Fill in the Blanks Type Questions
True False Type Questions
Short Answer Type Questions
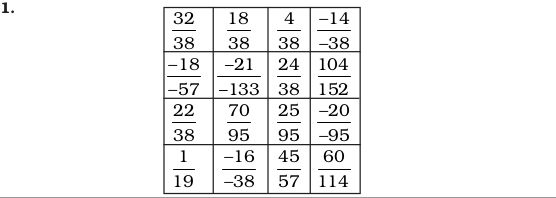
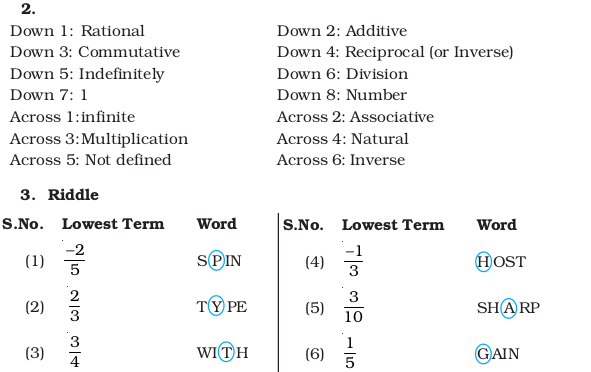
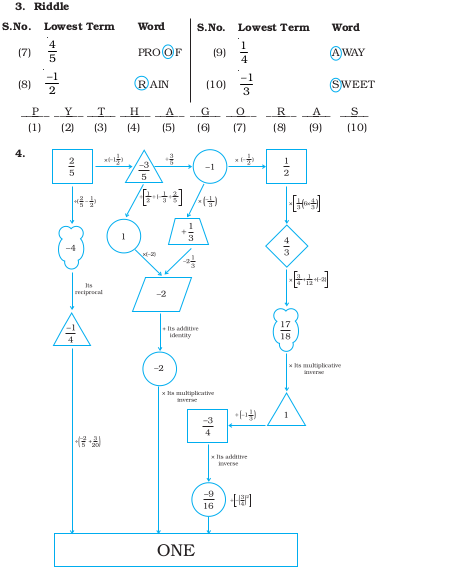
Games and Puzzles
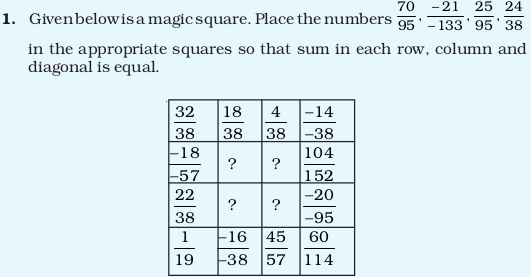
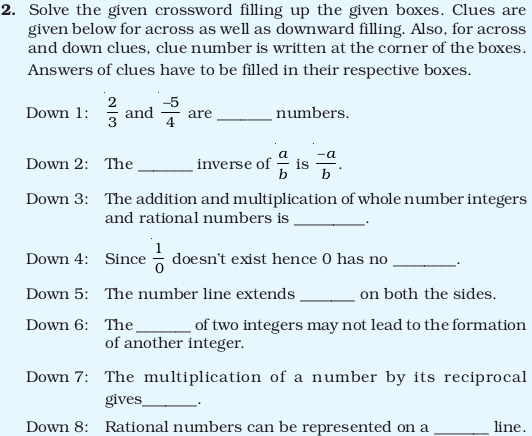
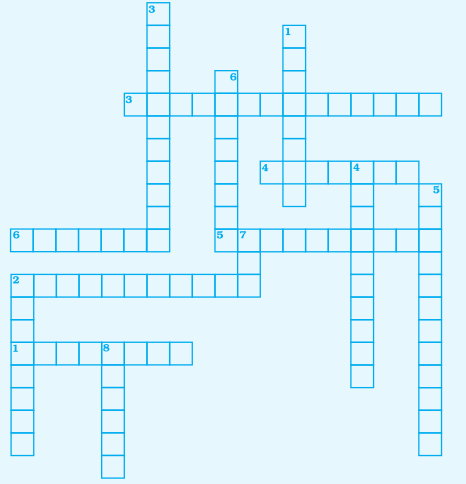
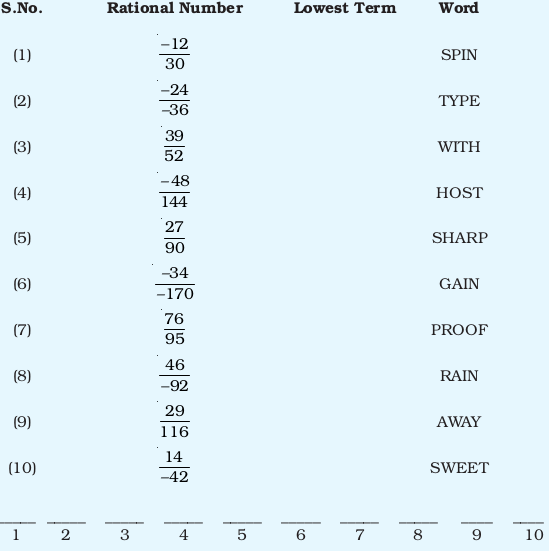
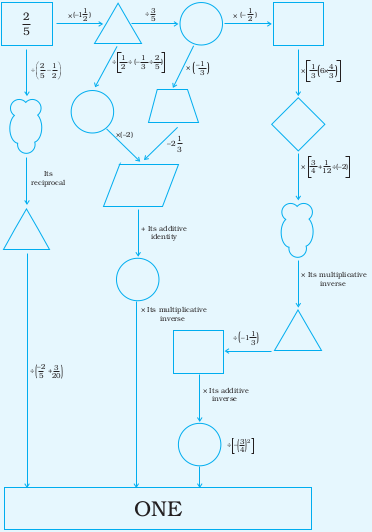
Click here to download NCERT Exemplar Class 8 Maths for Unit 1 Rational Numbers.
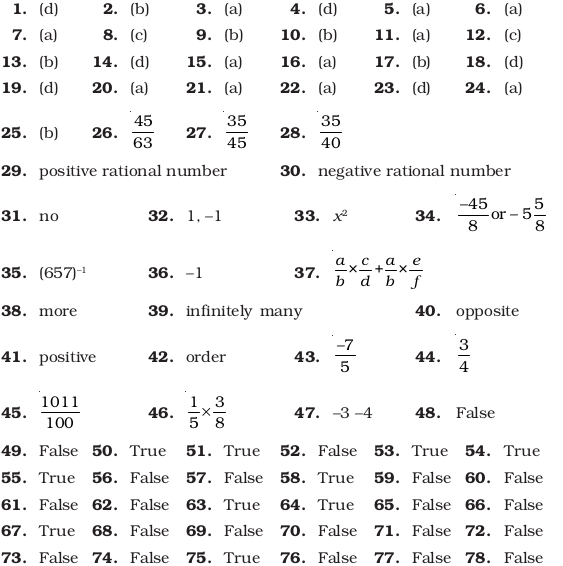
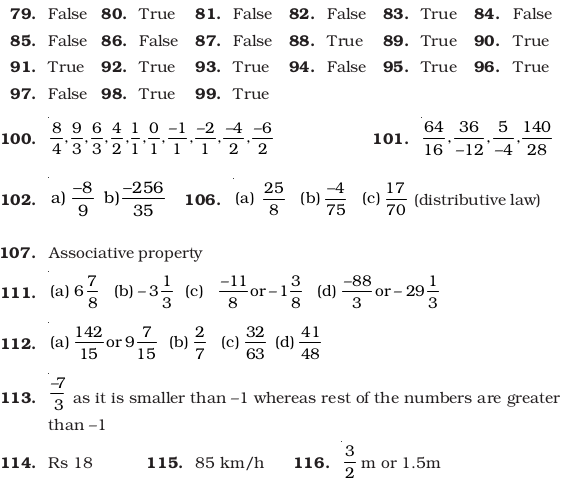
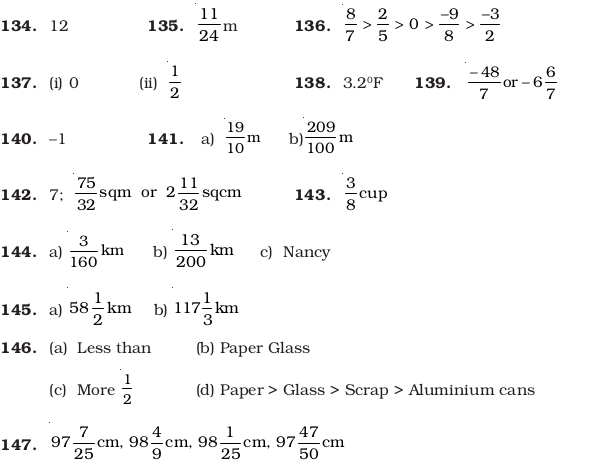
Answers to Multiple Choice Questions
Unit 1
Games and Puzzles
