NCERT Solutions for 12th Class Maths: Chapter 1-Relations and Functions

Class 12: Maths Chapter 1 solutions. Complete Class 12Maths Chapter 1 Notes.

NCERT Solutions for 12th Class Maths: Chapter 1-Relations and Functions

Class 12:Maths Chapter 1 solutions. Complete Class 12Maths Chapter 1 Notes.

Page No: 5

Exercise 1.1 (NCERT 12th Class Maths Chapter 1)

1. Determine whether each of the following relations are reflexive, symmetric and transitive:
(i) Relation R in the set A = {1, 2, 3, …, 13, 14} defined as R = {(x, y) : 3x – y = 0}

(ii) Relation R in the set N of natural numbers defined as R = {(x, y) : y = x + 5 and x < 4}

(iii) Relation R in the set A = {1, 2, 3, 4, 5, 6} as R = {(x, y) : y is divisible by x}

(iv) Relation R in the set Z of all integers defined as R = {(x, y) : x – y is an integer}

(v) Relation R in the set A of human beings in a town at a particular time given by
(a) R = {(x, y) : x and y work at the same place}
(b) R = {(x, y) : x and y live in the same locality}
(c) R = {(x, y) : x is exactly 7 cm taller than y}
(d) R = {(x, y) : x is wife of y}
(e) R = {(x, y) : x is father of y}

Answer

(i) A = {1,2,3,…..,13,14}
R = {x.y) : 3 x – y ≠} = {(x, y) : y = 3 x} = {(1,3), (2, 6), (3, 9), (4, 12)}

(a) R is not reflexive as (x, x) ∉ R    [ ∵ 3 x – x ≠ 0]

(b) R is not symmetric as (x,y) ∈ R does not imply (y, x) ∈ R
[∴ (1, 3) ∈ R does not imply (3. 1) ∈ R]

(c) R is not transitive as (1.3) ∈ R , (3, 9) ∈ R but (1.9) ∉ R

(ii) Relation R in the set N is given by
R = {(x, y) : y = x + 5 and x < 4}
∴ R = {(1,6), (2, 7). (3, 8)}

(a) R is not reflexive as (x, x) ∉ R

(b) R is not symmetric as (x, y) ∈ R ⇏ (v, x) ∈ R

(c) R is not transitive as (x,y) ∈ R, (y, z) ∈ R ⇏ (x, z) ∈ R

(iii) A = {1, 2, 3, 4, 5, 6}
R = {(x, y) : y is divisible by x}

(a) R is reflexive as (x, x) ∈ R ∀ x ∈ A    [∴ x divides x ∀ x ∈ A]

(b) R is not symmetric as (1, 6) ∈ R but (6, 1) ∉ R.

(c) Let (x, y), (y, z) ∈ A
∴ y is divisible by x and z is divisible by y
∴ z is divisible by x
∴ (r, y) ∈ R (y, z) ∈ R ⇒ (x, z) ∈ R
∴ R is transitive

(iv) Relation R is in the set Z given by R = {(x,y) : x – y is an integer}

(a) R is reflexive as ( x, x) ∈ R [∴ x – x = 0 is an integer]

(b) R is symmetric as (x,y) ∈ R ⇒ (y, x) ∈ A
[∵ x – y is an integer ⇒ y – x is an integer]

(c) R is transitive as (x, y), (y, z) ∈ R ⇒ (x, z) ∈ R
[∵ if x – y, y – z are integers, then (x – y) + (y – z) = x – z is also in integer]

(v) A is the set of human beings in a town at a particular time R is relation in A.

(a) R = {(x, y) : x and y work at the same time}
R is reflexive as (x, x) ∈ R

R is symmetric as ( x, y) ∈ R ∈ (y, x) ∈ R
[∵ x and y work at the same time ⇒ y and x work at the same time]

R is transitive as (x, y), (y, z) ∈ R ⇒ (x, z) ∈ R
[∴ if x and y, y and z work at the same time, then x and z also work at the same time]

(b) R = {(x,y) : x and y live in the same locality}
R is reflexive as (x, x) ∈ R

R is symmetric as ( x, y) ∈ R ⇒ (y, x) ∈ R
[∴ x and y live in the same locality ⇒ y and x live in the same locality]

R is transitive as ( x, y), ( y, z) ∈ R ⇒ (x, z) ∈ R
[∵ if x and y, y and z live in the same locality. then x and z also live in the same locality]

(c) R = {(x,y) : x is exactly 7 cm taller than y}
Since (x, x) ∉ R as x cannot be 7 cm taller than x.
∴ R is not reflexive.

(x, y) ∈ R ⇒ (y.x) ∈ R as if x is taller than y, then y cannot be taller than x.
∴ R is not symmetric.

Again, (x,y), (y,z) ∈ R ⇏ (x, z) ∈ R
[∵ if x is taller than y by 7 cm and y is taller than z by 7 cm, then x is taller than z by 14 cm]
∴ R is not transitive.

(d) R = {(x,y) : x is wife of y}
R is not reflexive as (x,y) ∉ R    [∴ x cannot be wife of x]

Also, (x, y) ∈ R ⇏ (y, x) ∈ R
[∵ if x is wife of y, then y cannot be wife of x]
∴ R is not symmetric.

 Also, this does not imply that x is the wife of z.
∴ (x, z) ∉ R
∴ R is not transitive.

(e) R = {(x,y) : x is father of y}
R is not reflexive as (x, x) ∉ R    [ ∵ x cannot be father of x]

Also, (x,y) ∈ R ⇐ (y, x) ∈ R [ ∵ if x is father of y. then y cannot be father of x]
∴ R is not symmetric.

Let (x, y) ∈ R and (y, z) ∈ R.
⇒ x is the father of y and y is the father of z.
⇒ x is not the father of z.
∴ x is the grandfather of z.
∴ (x, z) ∉ R
∴R is not transitive.

2. Show that the relation R in the set R of real numbers, defined as R = {(a, b) : a ≤ b²} is neither reflexive nor symmetric nor transitive.

Answer

R = {(a, b) : a ≤  b²}

(i) Since (a, a) ∉ R
[Take a = 1/3 then 1/3 > (1/3)²]
∴ R is not reflexive.

(ii) Also, (a, b) ∈ R ⇏ (b, a) ∈ R
[Take a = 2 ,b = 6, then 2 ≤ 62 but (6)2 < 2 is not true]
∴ R is not symmetric.

(iii) Now (a, b), (b, c) ∈ R ∉ (a, c) ∈ R
[Take a = 1, b = -2, c = -3 ∴ a ≤  b², b ≤ c² but a ≤ c² is not true]
∴ R is not transitive.

3. Check whether the relation R defined in the set {1, 2, 3, 4, 5, 6} as R = {(a, b) : b = a + 1} is reflexive, symmetric or transitive.

Answer

Let,
A = {1, 2, 3, 4, 5, 6}
R = {(a, b) : b = a + 1} = {(a, a + 1)}
= {(1, 2), (2, 3), (3, 4), (4,5), (5,6)}

(i) R is not reflexive as (a, a) ∉ R ∀ a ∈ A

(ii) (a,b) ∈ R ⇏ (b,a) ∈ R
 [∵ (a, b) ∈ R ⇒ b = a + 1 ⇒ a = b –1]
∴ R is not symmetric.

(iii) (a, b) ∈ R, (b, c) ∈ R ⇏ (a, c) ∈ R
[∵ (a, b), (b, c) ∈ R ⇒ b = a + 1, c = b + 1 ⇒ c = a + 2]
∴ R is not transitive.

4. Show that the relation R in R defined as R = {(a, b) : a ≤ b}, is reflexive and transitive but not symmetric.

Answer

R = {(a, b) : a ≤ b}

(i) Since (a, a) ∈ R ∀ a ∈ R
[∵ a ≤ a ∀ a ∈ R]
∴ R is reflexive.

(ii) (a, b) ∈ R ⇏ (b, a) ∈ R
[∵ if a ≤ b. then b ≤ a is not true]
∴ R is not symmetric.

(iii) Let (a, b), (b, c) ∈ R
∴ a ≤ b, b ≤ c
∴ a ≤ c ⇒ (a, c) ∈ R
∴ (a, b), (b. c) ∈ R ⇒ (a, c) ∈ R
∴ R is transitive.

5. Check whether the relation R in R defined by R = {(a, b) : a ≤ b³ } is reflexive, symmetric or transitive.

Answer

R = {(a, b) : a ≤ b³}

(i) Since (a, a) ∉ R as a ≤ a³ is not always true.
[Take a = 1/3. then a ≤ a³ is not true]
∴ R is not reflexive.

(ii) Also (a, b) ∈ R ⇏ (b, a) ∈ R
[Take a = 1, b = 4, ∴ 1 ≤ 4³ but 4 ≰ (l)³]
∴ R is not symmetric.

(iii) Now (a, b) ∈ R, (b, c) ∈ R ⇏ (a, c) ∈ R
[Take a = 100, b = 5, c = 3, ∴ 100 ≤ 5³, 5 ≤ 3³ but 100 ≥ 3³] R is not symmetric.

Page No: 6

6. Show that the relation R in the set{1, 2, 3} given by R={(1, 2), (2, 1)}is symmetric but neither reflexive nor transitive.

Answer

A = {1,2, 3}
R = {(1.2), (2, 1)}

Since, (a, a) ∈ R ∀ a ∈ A R is not reflexive.

Now (1, 2) ∈ R ⇒ (2, 1) ∈ R and (2, 1) ∈ R ⇒ (1,2) ∈ R
∴ (a, b) ∈ R ⇒ (b, a) ∈ R ∀ (a, b) ∈ R
∴ R is symmetric

Again, (1, 2) ∈ R and (2, 1) ∈ R but (1, 1) ∉ R
∴ R is not transitive.

7. Show that the relation R in the set A of all the books in a library of a college, given by R = {(x, y) : x and y have same number of pages} is an equivalence relation.

Answer

A is the set of all books in a library of a college.
R = {(x,y) : x and y have same number of pages}

Since, (x, x) ∈ R as x and x have the same number of pages ∀ x ∈ A.
∴ R is reflexive.

Also (x, y) ∈ R
⇒ x and y have the same number of pages
⇒ y and x have the same number of pages
⇒ (y, x) ∈ R
∴ R is symmetric.

Now, (x, y) ∈ R and (y, z) ∈ R.
⇒ x and y have the same number of pages and y and z have the same number of pages
⇒ x and z have he same number of pages
⇒ (x, z) ∈ R
∴ R is transitive.

8. Show that the relation R in the set A = {1, 2, 3, 4, 5} given by
R = {(a, b) : |a – b| is even}, is an equivalence relation. Show that all the elements of {1, 3, 5} are related to each other and all the elements of {2, 4} are related to each other. But no element of {1, 3, 5} is related to any element of {2, 4}.

Answer

A = {1, 2, 3, 4, 5}
R = {(a, b) : | a – b | is even}
Now | a – a | = 0 is an even number,
∴ (a, a) ∈ R ∀ a ∈ A
⇒ R is reflexive.

Again (a, b) ∈ R
⇒ | a – b | is even
⇒ | – (b – a) | is even
⇒ | b – a | is even
⇒ (b, a) ∈ R
∴ R is symmetric.

Let (a, b) ∈ R and (b, c) ∈ R
⇒ | a – b | is even and | b – c | is even
⇒ a – b is even and b – c is even
⇒ (a – b) + (b – c) is even
⇒ a – c is even ⇒ | a – c | is even
⇒ (a, c) ∈ R
∴ R is transitive.
∴ R is an equivalence relation.

∵ | 1 – 3 | = 2, | 3 – 5 | = 2 and | 1 – 5 | = 4 are even,
All the elements of {1, 3, 5} are related to each other.
∵ | 2 – 4 | = 2 is even,
All the elements of {2, 4} are related to each other.

Now | 1 – 2 | = 1, | 1 – 4 | = 3, | 3 – 2 | = 1, | 3 – 4 | = 1, | 5 – 2 | = 3 and | 5 – 4 | = 1 are all odd
No element of the set {1, 3, 5} is related to any element of (2, 4}.

9. Show that each of the relation R in the set A = {x ∈ Z : 0 ≤ x ≤ 12}, given by
(i) R = {(a, b) : |a – b| is a multiple of 4}
(ii) R = {(a, b) : a = b} is an equivalence relation.
Find the set of all elements related to 1 in each case.

Answer

A= {x ∈ Z : 0 ≤ x ≤ 12}
= {0, 1, 2, 3, 4, 5, 6, 7, 8,9, 10, 11, 12 }

(i) R = {(a, b) : | a – b | is a multiple of 4|
As | a – a | = 0 is divisible by 4
∴ (a, a) ∈ R ∀ a ∈ A.
∴ R is reflexive.

Next, let (a, b) ∈ R
⇒ | a – b | is divisible by 4

⇒ | – (b – a) | is divisible by 4
⇒ | b – a | is divisible by 4 ⇒ (b, a) ∈ R
∴ R is symmetric.

Again. (a, b) ∈ R and (b, c) ∈ R
⇒ |a – A| is a multiple of 4 and |b – c| is a multiple of 4
⇒ a – b is a multiple of 4 and b – c is a multiple of 4
⇒ (a – b) + (b – c) is a multiple of 4
⇒ a – c is a multiple of 4
⇒ |a – c| is a multiple of 4
⇒ (a, c) ∈ R
∴ R is transitive.
∴ R is an equivalence relation.
Set of elements which are related to | = {a ∈ A : (a, 1) ∈ R}
= {a ∈ A : |a – 1| is a multiple of 4]
= {1, 5, 9}
[ ∵ | 1 – 1 | = 0, | 5 – 1 | = 4 and I 9 – 1 | = 8 are multiples of 4] (ii) R = {(a, b) : a = b} ∴ a = a ∀ a ∈ A,

∴ R is reflexive.
Again, (a, b) ∈ R ⇒ a = b ⇒ b = a ⇒ (b,a) ∈ R ∴ R is symmetric.
Next. (a, b) ∈ R and (b, c) ∈ R
⇒    a = b and b = c
⇒    a = c ⇒ (a, c) ∈ R
∴ R is transitive.
∴ R is an equivalence relation.
Set of elements of A which are related to I = {a ∈ A : (a,1) ∈ R }
= {a ∈ A : a = 1} = {1}

10. Give an example of a relation. Which is
(i) Symmetric but neither reflexive nor transitive.
(ii) Transitive but neither reflexive nor symmetric.
(iii) Reflexive and symmetric but not transitive.
(iv) Reflexive and transitive but not symmetric.
(v) Symmetric and transitive but not reflexive.

Answer

(i) Let A = {1, 2}.
Then A x A = {(1,1), (1,2), (2,1), (2,2) }.
Let R = {(1,2), (2,1 )} .
Then R ⊆ A x A and hence R is a relation on the set A.
R is symmetric since (a, b) ∈ R ⇒ (b. a) ∈ R.
R is not reflexive since I ∈ A but (1,1) ∉ R.
R is not transitive since (1, 2) ∈ R, (2,1) ∈ R but (1,1) ∉ R.

(ii) Let A = {1,2,3}
Then A x A = {(1,1), (1,2), (1,3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3)}.
Let R = {(1,1), (2,2), (1,2), (2,1), (1,3), (2,3)}.
Then R is transitive since (a, b) ∈ R, (b, c) ∈ R ⇒ (a, c) ∈ R.
R is not reflexive since 3 G A but (3.3) ∉ R.
R is not symmetric since (1,3) ∈R but (3,1) ∉ R.

(iii) Let A = {1,2 3}
Then A x A = {(1, 1), (1,2), (1,3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3) }.
Let R = {(1,1), (2,2), (3,3), (1,2), (2,1), (2,3), (3,2)}.
R is a relation on A as R ⊆ A x A.
R is reflexive as (a, a) ∈ R ∀ a ∈ A.
Also. R is symmetric since (a. b) ∈ R implies that (b, a) ∈R.
But R is not transitive since (1,2) ∈R arid (2,3) ∈R but (1,3) ∢ R.

(iv) Let A = {1,2,3}.
Then A x A = {(1,1), (1,2), (1,3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3)}.
Let R = {(1,1), (2,2), (3,3), (1,3)}.
Then R is a relation on A as R ⊆ A x A.
R is reflexive since (a, a) ∈R ∀ a ∈ A.
R is not symmetric as (1,3) ∈R and (3,1) ∉ R. R is transitive since (a, b) ∈R and (b, c) ∈R implies that (a, c) ∈R.

(v) Let A = {1,2,3}
Then A x A = {(1,1), (1,2), (1, 3), (2,1), (2,2), (2,3), (3,1), (3,2), (3,3)}
Let R = {(1,1), (1,2), (2,1), (2,2)}.
R is not reflexive as 3∈ A and (3,3) ∉ R.
R is symmetric as (a, b) ∈ R ⇒ (b, a) ∈R.
R is transitive since (a, b) ∈ R and (b, c) ∈R implies that (a, c) ∈ R.

11. Show that the relation R in the set A of points in a plane given by R = {(P, Q) : distance of the point P from the origin is same as the distance of the point Q from the origin}, is an equivalence relation. Further, show that the set of all points related to a point P ≠ (0, 0) is the circle passing through P with origin as centre.

Answer

A is the set of points in a plane.
R = {(P. Q) : distance of the point P from the origin is same as the distance of the point Q from the origin}
= {(P, Q) : | OP | = | OQ | where O is origin}
Since, | OP | = | OP |, (P, P) ∈ R ∀ P ∈ A.
∴ R is reflexive.

Also (P. Q) ∈ R
⇒ | OP | = | OQ |
⇒ | OQ | = | OP |
⇒ (Q.P) ∈ R ⇒ R is symmetric.

Next let (P, Q) ∈ R and (Q, T) ∈ R ⇒     | OP | = | OQ | and | OQ | = | OT |
⇒ | OP | = | OT |
⇒ (P,T) ∈ R
∴ R is transitive

∴ R is an equivalence relation
Set of points related to P ≠ O
= {Q ∈ A : (Q,P) ∈ R} = {Q ∈ A : | OQ | = | OP |}
= {Q ∈ A :Q lies on a circle through P with centre O}

12. Show that the relation R defined in the set A of all triangles as R = {(T₁ , T₂) : T₁ is similar to T₂}, is equivalence relation. Consider three right angle triangles T₁ with sides 3, 4, 5, T₂ with sides 5, 12, 13 and T₃ with sides 6, 8, 10. Which triangles among T₁, T₂ and T₃ are related?

Answer

R = {(T₁, T₂) : T₁ is similar to T₂}
Since every triangle is similar to itself
∴ R is reflexive.
Also (T₁ T₂) ∈ R ⇒ T₁ is similar to T₂ ⇒ T₂ is similar to T₁
∴ (T₂,T₁) ⇒ R
∴ (T₁,T₂) ∈ R ⇒ (T₂,T₁) ∈ R ⇒ R is symmetric.
Again (T₁, T₂), (T₂, T₃) ∈ R
⇒ T₁ is similar to T₂ and T₂ is similar to T₃∴ T₁ is similar to T₃ ⇒ (T₁,T₃) ∈ R
∴ (T₁, T₂), (T₂,T₃) ∈ R ⇒ (T₁, T₃) ∈ R
∴ R is transitive.
∴ R is reflexive, symmetric and transitive
∴ R is an equivalence relation.
Now T₁, T₂, T₃ are triangles with sides 3, 4, 5 ; 5, 12, 13 and 6, 8, 10.
Since, 3/6 = 4/8 = 5/10
∴ T₁ is similar to T₃ i.e. T₃ is related to T₁.

13. Show that the relation R defined in the set A of all polygons as R = {(P₁, P₂) : P₁ and P₂ have same number of sides}, is an equivalence relation. What is the set of all elements in A related to the right angle triangle T with sides 3, 4 and 5?

Answer

A is the set of all polygons
R = {(P₁, P₂) : P₁ and P₂ have same number of sides }
Since  P₁ and P₂ have the same number of sides
∴ ( P₁, P₂) ∈ R ∀ P ∈ A.
∴ R is reflexive.

Let ( P₁, P₂) ∈ R
⇒  P₁ and  P₂ have the same number of sides ⇒  P₂ and P₁ have the same number of sides ⇒ ( P₂,  P₁) ∈ R
∴ (P₁, P₂) ∈ R ⇒ (P₂, P₁) ∈ R
∴ R is symmetric.

Let (P₁, P₂) ∈ R and (P₂, P₃) ∈ R.
⇒ P₁ and P₂ have the same number of sides and P₂ and P₃ have same number of sides
⇒ P₁ and P₃ have the same number of sides
⇒ (P₁, P₃) ∈ R
∴ (P₁, P₂), (P₂, P₃) ∈ R ∈ (P₁, P₃) ∈ R
∴ R is transitive.
∴ R is an equivalence relation.

Now T is a triangle.
Let P be any element of A.
Now P ∈ A is related to T iff P and T have the same number of sides P is a triangle required set is the set of all triangles in A.

14. Let L be the set of all lines in XY plane and R be the relation in L defined as R = {(L₁, L₂) : L₁ is parallel to L₂}. Show that R is an equivalence relation. Find the set of all lines related to the line y = 2x + 4.

Answer

R is reflexive because, in (L₁, L₁), L₁ and L₁ are parallel.
R is symmetric because, if L₁ and L₂ are parallel, then L₂ and L₁ are also parallel.
R is transitive because, if L₁ and L₂ are parallel; L₂ and L₃ are parallel, then L₁ and L₃ are also parallel.
Set of all lines parallel to y = 2x + 4 are all lines of the equation y = 2x + k , where k is any constant . (Two parallel lines will have the ratio of their x coefficients equal to that of y coefficients.).

Page No. 7

15. Let R be the relation in the set {1, 2, 3, 4} given by R = {(1, 2), (2, 2), (1, 1), (4,4), (1, 3), (3, 3), (3, 2)}. Choose the correct answer. 
(A) R is reflexive and symmetric but not transitive.
(B) R is reflexive and transitive but not symmetric. 
(C) R is symmetric and transitive but not reflexive. 
(D) R is an equivalence relation.

Answer

(A) R is reflexive and symmetric but not transitive
(B) R is reflexive and transitive but not symmetric
(C) R is symmetric and transitive but not reflexive.
(D) R is an equivalence relation.

R is reflexive because we have (1,1) (2,2) (3,3) and (4,4) in R.
R is not symmetric because (2,1), (3,1) doesn’t lie in R.
 R is transitive because (1,3) (3,2) (1,2) lie in R.

Thus option B is the correct answer

16. Let R be the relation in the set N given by R = {(a, b) : a = b – 2, b > 6}.
Choose the correct answer. 
(A) (2, 4) ∈ R  
(B) (3, 8) ∈ R 
(C) (6, 8) ∈ R 
(D) (8, 7) ∈ R

Answer

The correct option will C.

Option A is not possible, since b should be greater than 6.
Option B is not possible, since 3 = 8 – 2 is not possible.
Option C is possible , since 6 = 8 – 2
Option D is not possible, since 8 = 7 − 2 is not possible.

Page No: 10

Exercise 1.2 (NCERT 12th Class Maths Chapter 1)

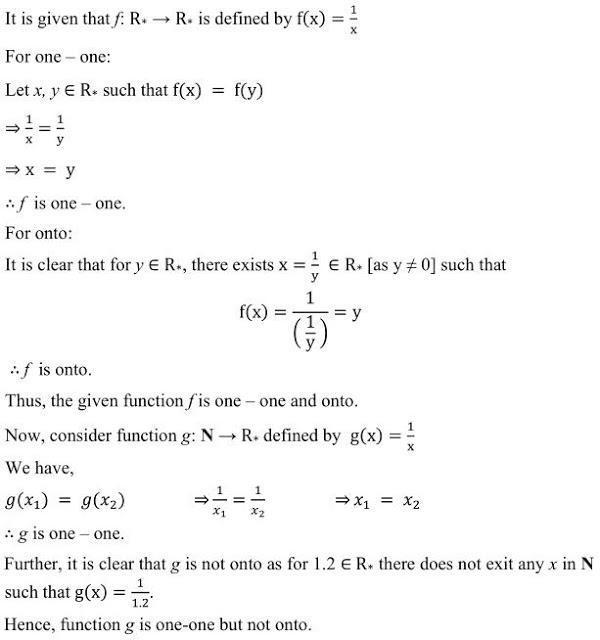
1. Show that the function f : R∗ → R∗ defined by f(x) = 1/x is one-one and onto, where R∗ is the set of all non-zero real numbers. Is the result true, if the domain R∗ is replaced by N with co-domain being same as R∗?

Answer

2. Check the injectivity and surjectivity of the following functions:

(i) f : N → N given by f(x) = x²

(ii) f : Z → Z given by f(x) = x² 

(iii) f : R → R given by f(x) = x²

(iv) f : N → N given by f(x) = x³

(v) f : Z → Z given by f(x) = x³ 

Answer

3. Prove that the Greatest Integer Function f : R → R, given by f(x) = [x], is neither one-one nor onto, where [x] denotes the greatest integer less than or equal to x.

Answer

f : R → R is given by, f(x) = [x]

It is seen that f(1.2) = [1.2] = 1

f(1.9)= [1.9] = 1

∴ f(1.2) = f(1.9), but 1.2 ≠ 1.9

∴ f is not one-one.

Now, consider 0.7 ∈ R.

It is known that f(x) = [x] is always an integer.

Thus, there does not exist any element x ∈ R such that f(x) = 0.7.

∴ f is not onto.

Hence, the greatest integer function is neither one-one nor onto.

Page No. 11

4. Show that the Modulus Function f : R → R, given by f(x) = |x|, is neither one-ne nor onto, where |x| is x, if x is positive or 0 and |x| is – x, if x is negative.

Answer

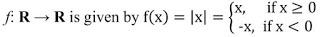
It is clear that f(-1) = I-1| = 1

and f(1) = I1l = 1

∴ f(-1) = f(1), but -1 ≠ 1

Now, consider -1 ∈ R.

∴ f is not one-one.

It is known that f(x) = Ix| is always non-negative.

Thus, there does not exist any element x in domain R such that f(x) = Ix| = -1. 

∴ f is not onto.

Hence, the modulus function is neither one-one nor onto.

5. Show that the Signum Function f : R → R, given by

Answer

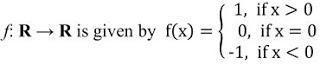
It is seen that f(1) = f(2) = 1, but 1  ≠ 2.

∴ f is not one-one.

Now, as f(x) takes only 3 values (1, 0, or -1) for the element -2 in co-domain R, there does not exist any x in domain R such that f(x) = -2. 

∴ f is not onto.

Hence, the Signum function is neither one-one nor onto.

6. Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. Show that f is one-one.

Answer

It is given that A= (1, 2, 3), B= (4, 5, 6, 7).

A → B is defined as f = {(1, 4), (2, 5), (3, 6)}

f(1)= 4,

f(2) = 5, 

f(3) = 6

It is seen that the images of distinct elements of A under f are distinct. Hence, function f is one-one. 

7. In each of the following cases, state whether the function is one-one, onto or bijective. Justify your answer.

(i) f : R → R defined by f(x) = 3 – 4x 

(ii) f : R → R defined by f(x) = 1+x²  

Answer

(i) f : R → 12 is defined as f(x) = 3 – 4x. 

Let x₁, x₂ ∈ R such that f(x₁) = f(x₂) 

⇒ 3-4x₁ = 3-4x₂

⇒ -4x₁ = -4x₂ 

⇒ x₁ = x₂ 

∴ f is one-one.

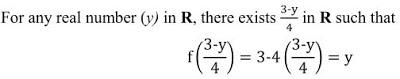
∴ f is onto.

Hence, f is bijective.

(ii) f: R → R is defined as f(x) = 1 + x2

Let x₁, x₂ ∈ R such that f(x₁) = f(x₂)

⇒ 1+ x₁² = 1 + x₂²

⇒ x₁² = x₂²

⇒ x₁ = ± x₂

∴ f(x₁) = f(x₂) does not imply that x₁ = x₂

8. Let A and B be sets. Show that f : A×B → B×A such that f(a, b) = (b, a) is bijective function.

Answer

f: A × B → B × A is defined as f(a, b) = (b, a).

Let (a₁, b₁), (a₂, b₂) ∈ A × B such that f(a₁, b₁) = f(a₂, b₂)

⇒ (b₁, a₁) = (b₂, a₂)

⇒ b₁ = b₂ and a₁ = a₂

⇒ (a₁, b₁) = (a₂, b₂)

∴ f is one – one.

Now, let (b, a) ∈ B × A be any element.

Then, there exists (a, b) ∈ A × B such that f(a, b) = (b, a). [By definition of f]

∴ f is onto.

Hence, f is bijective.

9. 

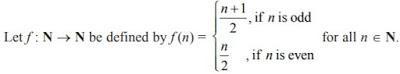
State whether the function f is bijective. Justify your answer.

Answer

10. Let A = R – {3} and B = R – {1}. Consider the function f : A → B defined by f(x) = (x-2)/(x-3).  Is f one-one and onto? Justify your answer.

Answer

11. Let f : R → R be defined as f(x) = x⁴. Choose the correct answer.

(A) f is one-one onto

(B) f is many-one onto

(C) f is one-one but not onto

(D) f is neither one-one nor onto.

Answer

f: R → R is defined as f(x) = x⁴

Let x, y ∈ R such that f(x) = f(y).

⇒ x⁴ = y⁴

⇒ x = ± y

∴ f(x) = f(y) does not imply that x = y.

For example f(1) = f(–1) = 1

∴ f is not one-one.

Consider an element 2 in co-domain R. It is clear that there does not exist any x in domain R such that f(x) = 2.

∴ f is not onto.

Hence, function f is neither one – one nor onto.

The correct answer is D.

12. Let f : R → R be defined as f(x) = 3x. Choose the correct answer.

(A) f is one-one onto 

(B) f is many-one onto 

(C) f is one-one but not onto 

(D) f is neither one-one nor onto.

Answer

f: R → R is defined as f(x) = 3x.

Let x, y ∈ R such that f(x) = f(y).

⇒ 3x = 3y

⇒ x = y

∴f is one-one.

Also, for any real number (y) in co-domain R, there exists y/3 in R such that f(y/3) = 3(y/3) = y

∴ f is onto.

Hence, function f is one – one and onto.

The correct answer is A.

Page No: 18

Exercise 1.3 (NCERT 12th Class Maths Chapter 1)

1. Let f : {1, 3, 4} → {1, 2, 5} and g : {1, 2, 5} → {1, 3} be given by f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}. Write down gof

Answer

The functions f: {1, 3, 4} → {1, 2, 5} and g: {1, 2, 5} → {1, 3} are defined as f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}.

gof(1) = g[f(1)] = g(2) = 3    [as f(1) = 2 and g(2) = 3]

gof(3) = g[f(3)] = g(5) = 1    [as f(3) = 5 and g(5) = 1]

gof(4) = g[f(4)] = g(1) = 3    [as f(4) = 1 and g(1) = 3]

∴ gof = {(1, 3), (3, 1), (4, 3)}

2. Let f, g and h be functions from R to R. Show that

(f + g) oh = foh + goh 

(f . g) oh = (foh) . (goh)

Answer

To prove: (f + g)oh = foh + goh

LHS = [(f + g)oh](x)

= (f + g)[h(x)]

= f [h(x)] + g[h(x)]

= (foh)(x) + (goh)(x)

= {(foh)(x) + (goh)}(x) = RHS

∴ {(f + g)oh}(x) = {(foh)(x) + (goh)}(x) for all x ∈R

Hence, (f + g)oh = foh + goh

To Prove: (f.g)oh = (foh).(goh)

LHS = [(f.g)oh](x)

= (f.g)[h(x)]

= f[h(x)] . g[h(x)]

= (foh)(x) . (goh)(x)

= {(foh).(goh)}(x) = RHS

∴ [(f.g)oh](x) = {(foh).(goh)}(x)    for all x ∈R

Hence, (f.g)oh = (foh).(goh)

3. Find gof and fog, if

(i) f(x) = | x | and g(x) = | 5x – 2 |

(ii) f(x) = 8x³ and g(x) = x^1/3.

Answer

(i). f(x) = |x| and g(x) = |5x-2|
∴gof(x) = g(f(x)) = g(|x|) = |5|x|-2|
fog(x) = f(g(x)) = f(|5x-2|) = ||5x-2|| = |5x-2|

(ii). f(x) = 8x³ and g(x) = x^1/3
∴gof(x) = g(f(x)) = g(8x³) = (8x³)^1/3 = 2x
fog(x) = f(g(x)) = f(x^1/3) = 8(x^1/3)³ = 8x

4. If f(x) = (4x+3)/(6x-4), x ≠ 2/3, show that fof(x) = x, for all x ≠ 2/3. What is the inverse of f ?

Answer

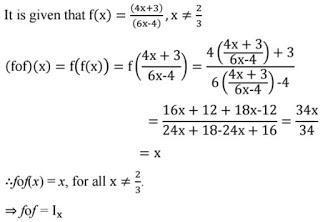
Hence, the given function f is invertible and the inverse of f is f itself.

5. State with reason whether following functions have inverse

(i) f : {1, 2, 3, 4} → {10} with

f = {(1, 10), (2, 10), (3, 10), (4, 10)}

(ii) g : {5, 6, 7, 8} → {1, 2, 3, 4} with

g = {(5, 4), (6, 3), (7, 4), (8, 2)}

(iii) h : {2, 3, 4, 5} → {7, 9, 11, 13} with

h = {(2, 7), (3, 9), (4, 11), (5, 13)}

Answer

(i) f: {1, 2, 3, 4} → {10} defined as f = {(1, 10), (2, 10), (3, 10), (4, 10)}

From the given definition of f, we can see that f is a many one function as f(1) = f(2) = f(3) = f(4) = 10

∴f is not one – one.

Hence, function f does not have an inverse.

(ii) g: {5, 6, 7, 8} → {1, 2, 3, 4} defined as g = {(5, 4), (6, 3), (7, 4), (8, 2)}

From the given definition of g, it is seen that g is a many one function as g(5) = g(7) = 4.

∴ g is not one – one.

Hence, function g does not have an inverse.

(iii) h: {2, 3, 4, 5} → {7, 9, 11, 13} defined as h = {(2, 7), (3, 9), (4, 11), (5, 13)}

It is seen that all distinct elements of the set {2, 3, 4, 5} have distinct images under h.

∴ Function h is one – one.

Also, h is onto since for every element y of the set {7, 9, 11, 13}, there exists an element x in the set {2, 3, 4, 5}, such that h(x) = y.

Thus, h is a one – one and onto function.

Hence, h has an inverse.

6. Show that f : [–1, 1] → R, given by f (x) =  x/(x+2)  is one-one. Find the inverse of the function f : [–1, 1] → Range f.

[Hint: For y ∈ Range f, y = f(x) =  x/(x+2), for some x in [–1, 1], i.e., x = 2y/(2y-1)]

Answer

f : [–1, 1] → R, given by f (x) =  x/(x+2)

For one-one,

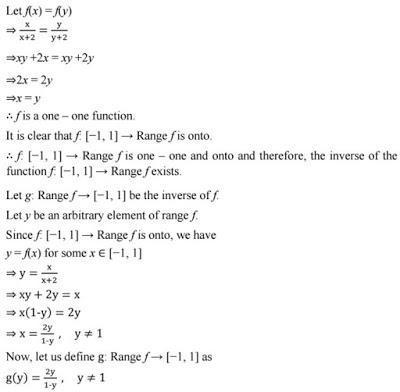
Now,

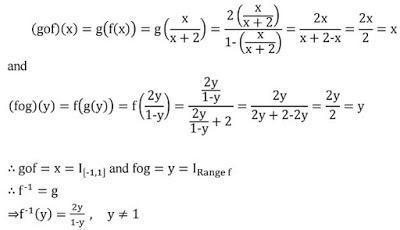
7. Consider f : R → R given by f(x) = 4x + 3. Show that f is invertible. Find the inverse of f.

Answer

f: R → R is given by, f(x) = 4x + 3

For one – one

Let f(x) = f(y)

⇒ 4x + 3 = 4y + 3

⇒ 4x = 4y

⇒ x = y

∴ f is a one – one function.

For onto

For y ∈ R, let y = 4x + 3.

⇒ x = y-3/4 ∈ R

Therefore, for any y ∈ R, there exists x = y-3/4 ∈ R, such that

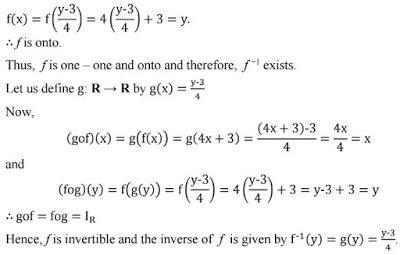
8. Consider f : R+ → [4, ∞) given by f(x) = x² + 4. Show that f is invertible with the inverse f^-1 of f given by f^-1(y) = √(y-4) , where R+ is the set of all non-negative real numbers.

Answer

f: R+ → [4, ∞) is given as f(x) = x² + 4.

For one – one

Let f(x) = f(y)

⇒ x² + 4 = y² + 4

⇒ x² = y²

⇒ x = y [as x = y ∈ R+]

∴ f is a one–one function.

For onto

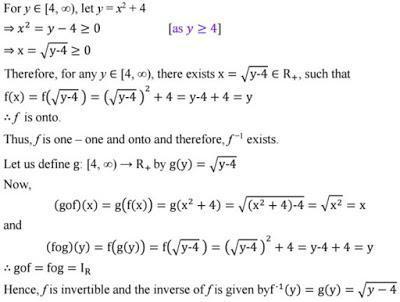
Page No. 19

9. Consider f : R+ → [– 5, ∞) given by f (x) = 9x² + 6x – 5. Show that f is invertible with 

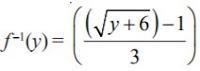
Answer

f: R+ → [−5, ∞) is given as f(x) = 9x² + 6x − 5.

Let y be an arbitrary element of [−5, ∞).

Let y = 9x² + 6x – 5

⇒ y = (3x+1)² -1 – 5 = (3x+1)² -6

⇒ y + 6 = (3x + 1)²

⇒ 3x + 1 = √(y + 6)

10. Let f : X → Y be an invertible function. Show that f has unique inverse.

(Hint: suppose g₁ and g₂ are two inverses of f. Then for all y ∈ Y, fog₁(y) = I_Y(y) = fog₂ (y). Use one-one ness of f).

Answer

Let f: X → Y be an invertible function.

Also, suppose f has two inverses (say g₁ and g₂)

Then, for all y∈Y, we have

fog₁(y) = I_Y(y) = fog₂(y)

⇒ f(g₁(y)) = f(g₂(y))

⇒ g₁(y) = g₂(y) [as f is invertible ⇒ f is one–one]

⇒ g₁ = g₂ [as g is one–one]

Hence, f has a unique inverse.

11. Consider f:{1, 2, 3} → {a, b, c} given by f(1) = a,f(2) = b and f(3) = c. Find f^-1 and show that (f^-1)^-1 = f.

Answer

Function f: {1, 2, 3} → {a, b, c} is given by f(1) = a, f(2) = b, and f(3) = c

If we define g: {a, b, c} → {1, 2, 3} as g(a) = 1, g(b) = 2, g(c) = 3.

We have,

(fog)(a) = f(g(a)) = f(1) = a

(fog)(b) = f(g(b)) = f(2) = b

(fog)(c) = f(g(c)) = f(3) = c

and

(gof)(1) = g(f(1)) = f(a) = 1

(gof)(2) = g(f(2)) = f(b) = 2

(gof)(3) = g(f(3)) = f(c) = 3

∴ gof = I_X and fog =  I_Y, where X = {1, 2, 3} and Y= {a, b, c}.

Thus, the inverse of f exists and f^-1 = g.

∴ f^-1:{a, b, c} → {1, 2, 3} is given by f^-1(a) = 1, f^-1(b) = 2, f^-1(c) = 3

Let us now find the inverse of f^-1 i.e., find the inverse of g.

If we define h: {1, 2, 3} → {a, b, c} as h(1) = a, h(2) = b, h(3) = c

We have

(goh)(1) = g(h(1)) = g(a) = 1

(goh)(2) = g(h(2)) = g(b) = 2

(goh)(3) = g(h(3)) = g(c) = 3

and

(hog)(a) = h(g(a)) = h(1) = a

(hog)(b) = h(g(b)) = h(2) = b

(hog)(c) = h(g(c)) = h(3) = c

∴ goh = I_X and hog = I_Y, where X = {1, 2, 3} and Y = {a, b, c}.

Thus, the inverse of g exists and g^-1 = h ⇒ (f^-1)^-1 = h.

It can be noted that h = f.

Hence, (f^-1)^-1 = f.

12. Let f: X → Y be an invertible function. Show that the inverse of f^-1 is f, i.e., (f^-1)^-1 = f

Answer

Let f: X → Y be an invertible function.
Then, there exists a function g: Y → X such that gof = I_X and fog = I_Y.
Here, f^-1 = g.
Now, gof = I_X and fog = I_Y
⇒ f^-1of = I_X and fof^-1 = I_Y
Hence, f^-1: Y → X is invertible and f is the inverse of f^-1
i.e., (f^-1)^-1 = f.

13. 13. If f: R → R be given by f(x) = ((3 – x³))^1/3, then fof(x) is
(a) x)^1/3
(b) x³
(c) x
(d) (3 – x³)

Answer

The correct option is C.

Page No: 24

Exercise 1.4

1. Determine whether or not each of the definition of ∗ given below gives a binary
operation. In the event that ∗ is not a binary operation, give justification for this.
(i) On Z⁺, define ∗ by a ∗ b = a – b
(ii) On Z⁺, define ∗ by a ∗ b = ab
(iii) On R, define ∗ by a ∗ b = ab²
(iv) On Z⁺, define ∗ by a ∗ b = |a – b|
(v) On Z⁺, define ∗ by a ∗ b = a

Answer

(i) On Z+, * is defined by a * b = a − b.

It is not a binary operation as the image of (1, 2) under * is 1 * 2 = 1 − 2 = −1 ∉ Z+

(ii) On Z+, * is defined by a * b = ab.

It is seen that for each a, b ∈ Z+, there is a unique element ab in Z+.

This means that * carries each pair (a, b) to a unique element a * b = ab in Z+.

Therefore, * is a binary operation.

(iii) On R, * is defined by a * b = ab².

It is seen that for each a, b ∈ R, there is a unique element ab² in R.

This means that * carries each pair (a, b) to a unique element a * b = ab² in R.

Therefore, * is a binary operation.

(iv) On Z+,* is defined by a * b = |a − b|.

It is seen that for each a, b ∈ Z+, there is a unique element |a − b| in Z+.

This means that * carries each pair (a, b) to a unique element a * b = |a − b| in Z+.

Therefore,* is a binary operation.

(v) On Z+,* is defined by a * b = a.

It is seen that for each a, b ∈ Z+, there is a unique element a in Z+.

This means that * carries each pair (a, b) to a unique element a * b = a in Z+.

Therefore, * is a binary operation.

2. For each operation ∗ defined below, determine whether ∗ is binary, commutative or associative.

(i) On Z, define a ∗ b = a – b 

(ii) On Q, define a ∗ b = ab + 1 

(iii) On Q, define a ∗ b = ab/2

(iv) On Z+ , define a ∗ b = 2^ab 

(v) On Z + , define a ∗ b = a^b

(vi) On R –{– 1}, define a ∗ b = a/b+1

Answer

(i) On Z, * is defined by a * b = a − b.
It can be observed that 1 * 2 = 1 − 2 = −1 and 2 * 1 = 2 − 1 = 1.
∴1 * 2 ≠ 2 * 1, where 1, 2 ∈ Z
Hence, the operation * is not commut
Also, we have
(1 * 2) * 3 = (1 − 2) * 3 = −1 * 3 = −1 − 3 = −4
1 * (2 * 3) = 1 * (2 − 3) = 1 * −1 = 1 − (−1) = 2
∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Z
Hence, the operation * is not associative.

(ii) On Q, * is defined by a * b = ab + 1.
It is known that: ab = ba for all a, b ∈ Q
⇒ ab + 1 = ba + 1 for all a, b ∈ Q
⇒ a * b = a * b for all a, b ∈ Q
Therefore, the operation * is commutative.
It can be observed that
(1 * 2) * 3 = (1 × 2 + 1) * 3 = 3 * 3 = 3 × 3 + 1 = 10
1 * (2 * 3) = 1 * (2 × 3 + 1) = 1 * 7 = 1 × 7 + 1 = 8
∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Q
Therefore, the operation * is not associative.

(iii) On Q, * is defined by a * b =ab/2
It is known that: ab = ba for all a, b ∈ Q
⇒ab/2 = ba/2
for all a, b ∈ Q
⇒ a * b = b * a for all a, b ∈ Q
Therefore, the operation * is commutative.
For all a, b, c ∈ Q, we have

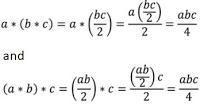
∴ (a*b)*c = a*(b*c), where a, b, c ∈ Q

Therefore, the operation * is associative.

(iv) On Z+, * is defined by a * b = 2^ab.

It is known that: ab = ba for all a, b ∈ Z+

⇒ 2^ab  = 2^ba for all a, b ∈ Z+

⇒ a * b = b * a for all a, b ∈ Z+

Therefore, the operation * is commutative.

It can be observed that

(1 ∗ 2) ∗ 3 = 2^1×2 ∗ 3 = 4 ∗ 3 =2^4×3 = 2¹² and

1 ∗ (2 ∗ 3) = 1 ∗ 2^2×3 = 1 ∗ 2⁶ = 1 ∗ 64 = 2^1×64 = 2⁶⁴

∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Z+

Therefore, the operation * is not associative.

(v) On Z+, * is defined by a * b = a^b.

It can be observed that

1*2 = 1² = 1 and 2*1 = 2¹ = 2

∴ 1 * 2 ≠ 2 * 1, where 1, 2 ∈ Z+

Therefore, the operation * is not commutative.

It can also be observed that

(2 ∗ 3) ∗ 4 = 2³ ∗ 4 = 8 ∗ 4 = 8⁴ = 2¹² and

2 ∗ (3 ∗ 4) = 2 ∗ 3⁴ = 2 ∗ 81 = 2⁸¹

∴ (2 * 3) * 4 ≠ 2 * (3 * 4), where 2, 3, 4 ∈ Z+

Therefore, the operation * is not associative.

(vi) On R, * −{−1} is defined by a ∗ b = a/b+1

It can be observed that

1 ∗ 2 = 1/2+1 = 1/3 and 2 ∗ 1 = 2/1+1 = 2/2 = 1

∴1 * 2 ≠ 2*1, where 1, 2 ∈ R − {−1}

Therefore, the operation * is not commutative.

It can also be observed that

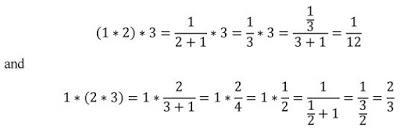
∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ R −{−1}

Therefore, the operation * is not associative.

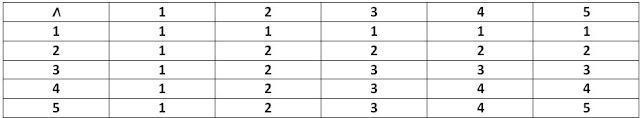
3. Consider the binary operation ∧ on the set {1, 2, 3, 4, 5} defined by a ∧ b = min {a, b}. Write the operation table of the operation ∧.

Answer

The binary operation ∧ on the set {1, 2, 3, 4, 5} is defined as a ∧ b = min {a, b} for all a, b ∈ {1, 2, 3, 4, 5}.

Thus, the operation table for the given operation ∧ can be given as:

Page No. 25

4. Consider a binary operation ∗ on the set {1, 2, 3, 4, 5} given by the following multiplication table (Table 1.2).

(i) Compute (2 ∗ 3) ∗ 4 and 2 ∗ (3 ∗ 4)

(ii) Is ∗ commutative?

(iii) Compute (2 ∗ 3) ∗ (4 ∗ 5). (Hint: use the following table)

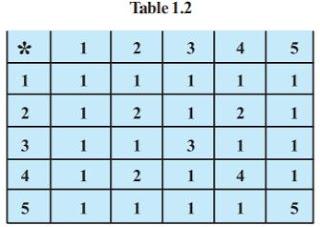
Answer

(i) (2 * 3) * 4 = 1 * 4 = 1

2 * (3 * 4) = 2 * 1 = 1

(ii) For every a, b ∈ {1, 2, 3, 4, 5}, we have a * b = b * a.

Therefore, the operation * is commutative.

(iii) (2 * 3) = 1 and (4 * 5) = 1

∴ (2 * 3) * (4 * 5) = 1 * 1 = 1

5. Let ∗′ be the binary operation on the set {1, 2, 3, 4, 5} defined by a ∗′ b = H.C.F. of a and b. Is the operation ∗′ same as the operation ∗ defined in Exercise 4 above? Justify your answer.

Answer

The binary operation *′ on the set {1, 2, 3 4, 5} is defined as a *′ b = H.C.F of a and b.

The operation table for the operation *′ can be given as:

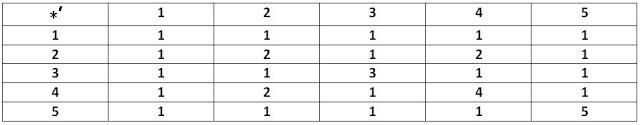
We observe that the operation tables for the operations * and *′ are the same.

Thus, the operation *′ is same as the operation*.

6. Let ∗ be the binary operation on N given by a ∗ b = L.C.M. of a and b. Find

(i) 5 ∗ 7, 20 ∗ 16

(ii) Is ∗ commutative?

(iii) Is ∗ associative?

(iv) Find the identity of ∗ in N

(v) Which elements of N are invertible for the operation ∗?

Answer

The binary operation * on N is defined as a * b = L.C.M. of a and b.

(i) 5 * 7 = L.C.M. of 5 and 7 = 35

20 * 16 = L.C.M of 20 and 16 = 80

(ii) It is known that

L.C.M of a and b = L.C.M of b and a for all a, b ∈ N.

∴ a * b = b * a

Thus, the operation * is commutative.

(iii) For a, b, c ∈ N, we have

(a * b) * c = (L.C.M of a and b) * c = LCM of a, b, and c

a * (b * c) = a * (LCM of b and c) = L.C.M of a, b, and c

∴ (a * b) * c = a * (b * c)

Thus, the operation * is associative.

(iv) It is known that:

L.C.M. of a and 1 = a = L.C.M. 1 and a for all a ∈ N

⇒ a * 1 = a = 1 * a for all a ∈ N

Thus, 1 is the identity of * in N.

(v) An element a in N is invertible with respect to the operation * if there exists

an element b in N, such that a * b = e = b * a.

Here, e = 1

This means that

L.C.M of a and b = 1 = L.C.M of b and a

This case is possible only when a and b are equal to 1.

Thus, 1 is the only invertible element of N with respect to the operation *.

7. Is ∗ defined on the set {1, 2, 3, 4, 5} by a ∗ b = L.C.M. of a and b a binary operation? Justify your answer.

Answer

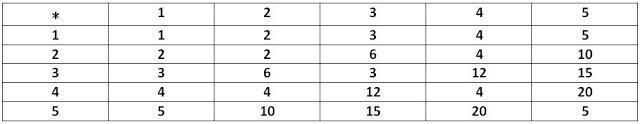
The operation * on the set A = {1, 2, 3, 4, 5} is defined as a * b = L.C.M. of a and b.

Then, the operation table for the given operation * can be given as:

It can be observed from the obtained table that

3 * 2 = 2 * 3 = 6 ∉ A,

5 * 2 = 2 * 5 = 10 ∉ A,

3 * 4 = 4 * 3 = 12 ∉ A,

3 * 5 = 5 * 3 = 15 ∉ A,

4 * 5 = 5 * 4 = 20 ∉ A

Hence, the given operation * is not a binary operation.

8. Let ∗ be the binary operation on N defined by a ∗ b = H.C.F. of a and b.

Is ∗ commutative? Is ∗ associative? Does there exist identity for this binary operation on N?

Answer

The binary operation * on N is defined as: a * b = H.C.F. of a and b

It is known that

H.C.F. of a and b = H.C.F. of b and a for all a, b ∈ N.

∴ a * b = b * a

Thus, the operation * is commutative.

For a, b, c ∈ N, we have

(a * b)* c = (H.C.F. of a and b) * c = H.C.F. of a, b and c

a *(b * c) = a *(H.C.F. of b and c) = H.C.F. of a, b, and c

∴ (a * b) * c = a * (b * c)

Thus, the operation * is associative.

Now, an element e ∈ N will be the identity for the operation * if a * e = a = e* a for all a ∈ N.

But this relation is not true for any a ∈ N.

Thus, the operation * does not have any identity in N.

9. Let ∗ be a binary operation on the set Q of rational numbers as follows:

(i) a ∗ b = a – b

(ii) a ∗ b = a² + b²

(iii) a ∗ b = a + ab

(iv) a ∗ b = (a – b)²

(v) a ∗ b = ab/4

(vi) a ∗ b = ab²

Answer

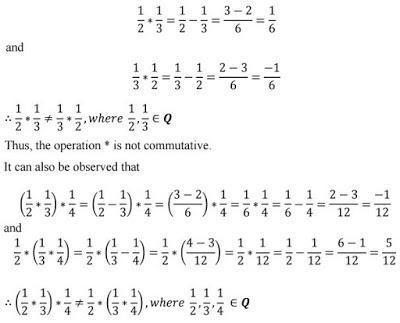
(i) On Q, the operation * is defined as a * b = a − b. It can be observed that:

Thus, the operation * is not associative.

(ii) On Q, the operation * is defined as a * b = a² + b².

For a, b ∈ Q, we have

a * b = a² + b² = b² + a² = b * a

∴ a * b = b * a

Thus, the operation * is commutative.

It can be observed that

(1 * 2) * 3 = (1² + 2²) * 3 = (1 + 4) * 3 = 5 * 3 = 5² + 3² = 3⁴ and

1 * (2 * 3) = 1 * (2² + 3²) = 1 * (4 + 9) = 1 * 13 = 1² + 13² =170

∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Q

Thus, the operation * is not associative.

(iii) On Q, the operation * is defined as a * b = a + ab.

It can be observed that

1 * 2 = 1 + 1×2 = 1 + 2 = 3

2 * 1 = 2 + 2×1 = 2 + 2 = 4

∴ 1 * 2 ≠ 2 * 1, where 1, 2 ∈ Q

Thus, the operation * is not commutative.

It can also be observed that

(1 * 2) * 3 = (1+ 1×2) * 3 = (1 + 2) * 3 = 3 * 3 = 3 + 3×3 = 3 + 9 = 12 and

1 * (2 * 3) = 1 * (2 + 2×3 ) = 1 * (2 + 6) = 1 * 8 = 1 + 1×8 =1 + 8 = 9

∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Q

Thus, the operation * is not associative.

(iv) On Q, the operation * is defined by a * b = (a − b)².

For a, b ∈ Q, we have

a * b = (a − b)²

b * a = (b − a)² = [− (a − b)]² = (a − b)²

∴ a * b = b * a

Thus, the operation * is commutative.

It can be observed that

(1 * 2) * 3 = (1 – 2)² * 3 = (-1)² * 3 = 1 * 3 = (1 – 3)² = (-2) = 4 and

1 * (2 * 3) = 1 * (2 – 3)² = 1 * (-1)² = 1 * 1 = (1 – 1)² = 0

∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Q

Thus, the operation * is not associative.

(v) On Q, the operation * is defined as a*b = ab/4.

For a, b ∈ Q, we have a*b = ab/4 = ba/4 = b*a

∴ a * b = b * a

Thus, the operation * is commutative.

For a, b, c ∈ Q, we have

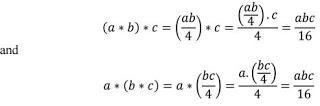
∴ (a * b) * c = a * (b * c), where a, b, c ∈ Q

Thus, the operation * is associative.

(vi) On Q, the operation * is defined as a * b = ab²

It can be observed that

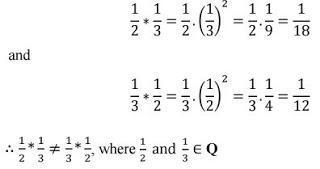
∴ 1/2 * 1/3 ≠ 1/3 * 1/2, where 1/2 and 1/3 ∈ Q

Thus, the operation * is not commutative.

It can also be observed that

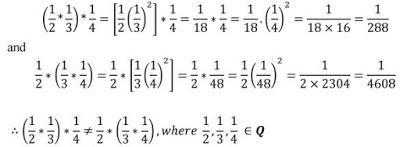
Thus, the operation * is not associative. 

Hence, the operations defined in (ii), (iv), (v) are commutative and the operation defined in (v) is associative.

10. Find which of the operations given above has identity.

Answer

An element e ∈ Q will be the identity element for the operation *

if a * e = a = e * a, for all a ∈ Q.

However, there is no such element e ∈ Q with respect to each of the six operations satisfying the above condition.

Thus, none of the six operations has identity.

11. Let A = N × N and ∗ be the binary operation on A defined by

(a, b) ∗ (c, d) = (a + c, b + d)

11. Show that ∗ is commutative and associative. Find the identity element for ∗ on A, if any.

Answer

A = N × N and * is a binary operation on A and is defined by

(a, b) * (c, d) = (a + c, b + d)

Let (a, b), (c, d) ∈ A

Then, a, b, c, d ∈ N

We have:

(a, b) * (c, d) = (a + c, b + d)

(c, d) * (a, b) = (c + a, d + b) = (a + c, b + d)

[Addition is commutative in the set of natural numbers]

∴ (a, b) * (c, d) = (c, d) * (a, b)

Therefore, the operation * is commutative.

Now, let (a, b), (c, d), (e, f) ∈ A

Then, a, b, c, d, e, f ∈ N

We have

[(a, b) ∗ (c, d)] ∗ (e, f) = (a + c, b + d) ∗ (e, f) = (a + c + e, b + d + f)

and

(a, b) ∗ [(c, d) ∗ (e, f)] = (a, b) ∗ (c + e, d + f) = (a + c + e, b + d + f)

∴ [(a, b) ∗ (c, d)] ∗ (e, f) = (a, b) ∗ [(c, d) ∗ (e, f)]

Therefore, the operation * is associative.

Let an element e = (e₁, e₂) ∈ A will be an identity element for the operation *

if a * e = a = e * a for all a = (a₁, a₂) ∈ A

i.e., (a₁ + e₁, a₂ + e₂) = (a₁, a₂) = (e₁ + a₁, e₂ + a₂)

Which is not true for any element in A.

Therefore, the operation * does not have any identity element.

Page No. 26

12. State whether the following statements are true or false. Justify.

(i) For an arbitrary binary operation ∗ on a set N, a ∗ a = a ∀ a ∈ N.

(ii) If ∗ is a commutative binary operation on N, then a ∗ (b ∗ c) = (c ∗ b) ∗ a

Answer

(i) Define an operation * on N as a * b = a + b ∀ a, b ∈ N

Then, in particular, for b = a = 3, we have

3 * 3 = 3 + 3 = 6 ≠ 3

Therefore, statement (i) is false.

(ii) R.H.S. = (c * b) * a

= (b * c) * a [* is commutative]

= a * (b * c) [Again, as * is commutative]

= L.H.S.

∴ a * (b * c) = (c * b) * a

Therefore, statement (ii) is true.

13. Consider a binary operation ∗ on N defined as a ∗ b = a³ + b³. Choose the correct answer.

(A) Is ∗ both associative and commutative?

(B) Is ∗ commutative but not associative?

(C) Is ∗ associative but not commutative?

(D) Is ∗ neither commutative nor associative?

Answer

On N, the operation * is defined as a * b = a3 + b3.

For, a, b, ∈ N, we have

a * b = a³ + b³ = b³ + a³ = b * a [Addition is commutative in N]

Therefore, the operation * is commutative.

It can be observed that

(1 * 2) * 3 = (1³ + 2³) * 3 = (1 + 8) * 3 = 9 * 3 = 9³ + 3³ = 729 + 27 = 756

and 1* (2 * 3) = 1 * (2³ + 3³) = 1* (8 + 27) = 1*35 = 1³ + 35³ = 1 + 42875 = 42876

∴ (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ N

Therefore, the operation * is not associative.

Hence, the operation * is commutative, but not associative.

Thus, the correct answer is B.

Class 12:Maths Chapter 1 solutions. Complete Class 12Maths Chapter 1 Notes.

NCERT Solutions for 12th Class Maths: Chapter 1: Download PDF

NCERT Solutions for 12th Class Maths: Chapter 1-Relations and Functions

Download PDF: NCERT Solutions for 12th Class Maths: Chapter 1-Relations and Functions PDF

Chapterwise NCERT Solutions for Class 12 Maths :

• Chapter 1 – Relations and Functions
• Chapter 2 – Inverse Trigonometric Functions.
• Chapter 3 – Matrices
• Chapter 4 – Determinants.
• Chapter 5 – Continuity and Differentiability.0.0
• Chapter 6 – Application of Derivatives.
• Chapter 7 – Integrals.
• Chapter 8 – Application of Integrals.
• Chapter 9: Differential Equations
• Chapter 10: Vector Algebra
• Chapter 11: Three Dimensional Geometry
• Chapter 12: Linear Programming
• Chapter 13: Probability

About NCERT

The National Council of Educational Research and Training is an autonomous organization of the Government of India which was established in 1961 as a literary, scientific, and charitable Society under the Societies Registration Act. Its headquarters are located at Sri Aurbindo Marg in New Delhi. Visit the Official NCERT website to learn more.